Path Planning

Lib5K provides a few types of path generation tools, mainly: Path and SmoothPath. These tools are used for converting a small list of control points (aka. waypoints) into a longer list of closely spaced goal poses for the robot to follow.

Path

The Path class creates a linear, point-to-point path from a few control points:

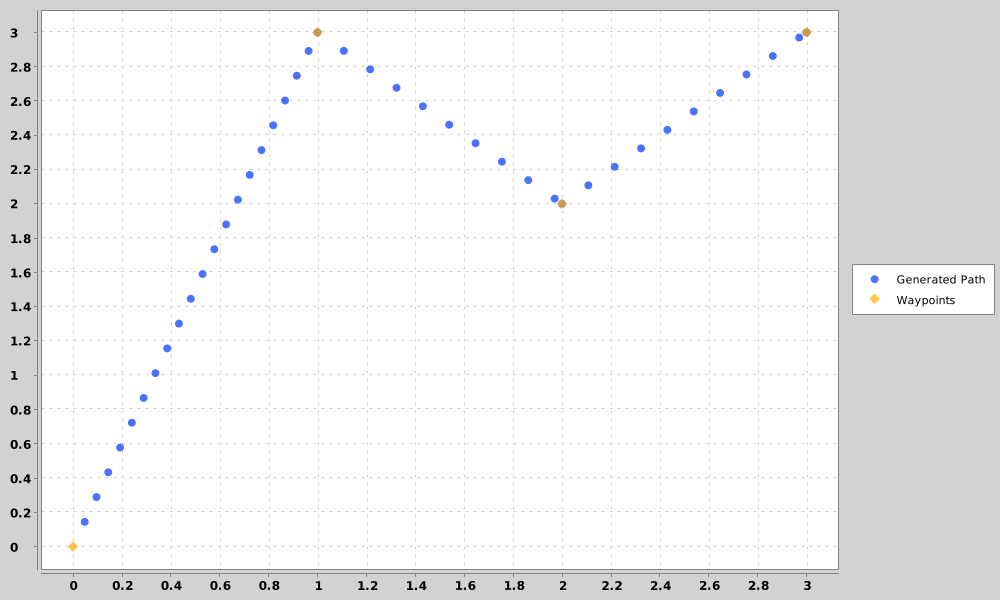
The process for generating the inner points (blue) is as follows. For every pair of control points in the path, we can get their magnitude with (where \(\vec{b}\) is the first control point, and \(\vec{e}\) is the second):

\[\begin{aligned} \vec{d} &= \vec{e} - \vec{b} \\ \lVert m \rVert &= \sqrt{\vec{d}_0^2 + \vec{d}_1^2} \end{aligned}\]

and get their unit vector with:

\[\vec{u} = \frac{\vec{d}}{m}\]

To determine the number of inner points that are required between the two control points, we simply divide the magnitude by a spacing (\(s\)) constant (we almost always use 6 inches for this value). Where \(\vec{P_{n-1}}\) is the previously calculated inner point, we can place the next inner point (\(\vec{P_n}\)) with:

\[\vec{P_n} = \vec{P_{n-1}} + \vec{u}s\]

Continuing this process through all control points, we get a linear path.

(Java implementation is here)

SmoothPath

The SmoothPath class extends Path, as it takes a linear, point-to-point path and modifies it using a set number of erosion steps.

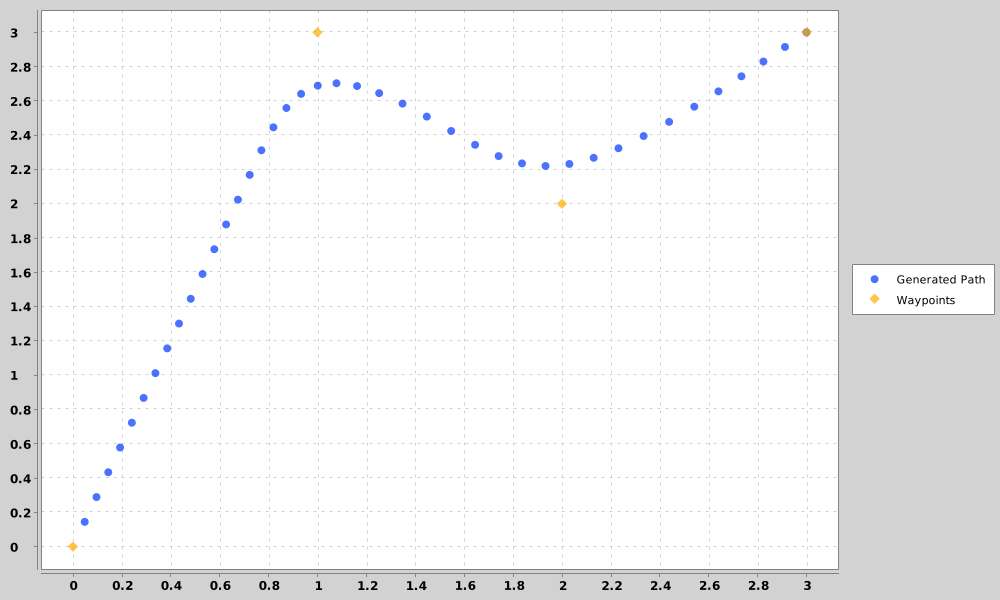
One cycle of the erosion step involves running computing the following for every inner point in the path. Cycling multiple times will improve the smoothness of the path. When eroding a path, a copy is made, so there is a list of origional, un-modified points, and a list of modified points (the inner points being modified by the erosion step). In the following equations, \(\vec{P_n}\) is the current un-modified inner point, \(\vec{A_n}\) is the current modified inner point. Like above, the subscripts n-1 and n+1 refer to the previous and next points. \(w_1\) and \(w_2\) are coefficients that affect the aggression of each erosion step.

\[\vec{A_n} = \vec{A_n} + w_1(\vec{P_n} - \vec{A_n}) + w_2(\vec{A_{n-1}} + \vec{A_{n+1}} - 2\vec{A_n})\]

The above visualization uses 0.5 as both the \(w_1\) and \(w_2\) values.

(Java implementation is here)